Every time the Powerball Lottery breaks two-hundred million or so, people at work start talking about it and emailing appeals for joining a lotto pool. After I got sick of shaking my head at these people, I realized that it could possibly be worth it to buy a ticket. Since the ticket price stays the same even as the jackpot grows, there’s got to be a tipping point, beyond which the payoff from the average ticket is greater than one dollar. But what is that point? Should I be queuing up at the gas station right now? Or should I wait until it hits ten digits?
Powerball tells you that the odds of getting the grand prize are 1 in 146,107,962. This gives us a good starting point. In a universe without taxes, annuties or interest, a rational person wouldn’t want to purchase a ticket until the jackpot was $146 million. But we don’t live in that universe, nor do I believe we’ll be travelling to it to play the lottery.
To figure out the answer for us, we’ll need to start by answering one quite difficult question: When they say “estimated jackpot of” whatever, how much of that is going to end up directly in your pocket? The Powerball FAQ talks a bit about how the payoff works (towards the bottom). The advertised estimated jackpot is the annuity, and it’s usually about double the actual cash they have on-hand. If you go for the lump-sum choice, you get that amount of cash (this week, about $150 million). Interestingly, if you choose the annuity, they put that same pile of money into government bonds, and they pay you in 30 yearly installments, funded entirely from that investment.
This detail is important for two reasons. First, even if you buy the same bonds the Powerball folks would have (which you can’t, since you’re a nobody schlub who thought the lottery was a decent investment decision), you end up with a lot less money by picking the lump sum. They’re able to buy the bonds without paying income taxes — which means far more interest. Since it’s income for you even before you can buy anything with it, you aren’t so lucky. Second, they don’t say “estimated jackpot” because they’re lazy; they say it because it’s impossible for anyone to say how bonds are going to behave for the next 30 years. They couldn’t tell you how much money you’ll end up getting if they wanted to. Which they probably don’t.
Unfortunately, this also makes things impossible for us to calculate accurately. So we’re going to have to be estimated it about it as well. So let’s say their double-the-lump-sum (L = J/2) guess is accurate. Accepting the annuity, you end up with J/30 per year. This is (obviously) going to put you in the top tax bracket: 35%. Which means that only about (1 - 0.35) * (J/30) is yours, per year. A little simple arithmetic brings us to the conclusion that J must be at least $224 million.
But what have I forgotten? I’ll give you a moment to consider what I’ve looked at so far. Hundreds of millions of dollars. Decades of interest. A dope with a little slip of paper and Hurley’s numbers. Got it, yet? It’s Carter’s Bane, better known as inflation. The Department of Labor follows inflation using the Consumer Price Index, a complicated formula of a whole bunch of items that pretty much everyone pays for: food, gas, housing, clothes, silly hats, medical care, etc. Looking at a summary table of CPI indexes, the average inflation rate for the past decade has been just over 2.5% year to year.
What does this mean? It means that in 2036, when you’re getting your very last annuity, although it’s (more or less) exactly the same number of dollars, it’ll be worth less than half as much as your first one ((1-2.5%)^29 = 0.48). And your total? Knowing that we want $146 million at the end, we can calculate for J in Σ(n=[0,29]) of ((1-2.5%)^n)((1 - 0.35)(J/30)), and we get a final answer of $316,822,280.
Don’t run out to buy any tickets right now. But if no one wins tonight, you might want to get some for next Saturday’s drawing.
Update, 16 Feb: Last night, mulling this over, I decided that the probabilities of winning non-jackpot prizes were significant enough to consider. Also, I decided that I was calculating from the wrong direction. Knowing (almost) all of the outcome values and probabilities, it should be easy to setup an equation for the value of the average ticket and solve for when that value equals one dollar. It was easy:
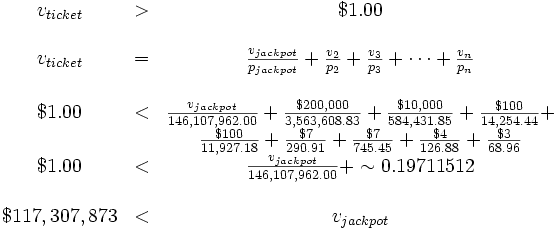
So when the pocket value of the jackpot is over $117 million, a ticket is worth more than it costs. The pocket value is equal to the inflation-corrected post-taxes estimate we made above (about 46.117% of the advertised estimation). An estimated jackpot of about $254 million would satisfy these conditions. No one won last night, and they're advertising above $360 million for Saturday.